What Does RMS Stand For: Exploring the Meaning and Significance

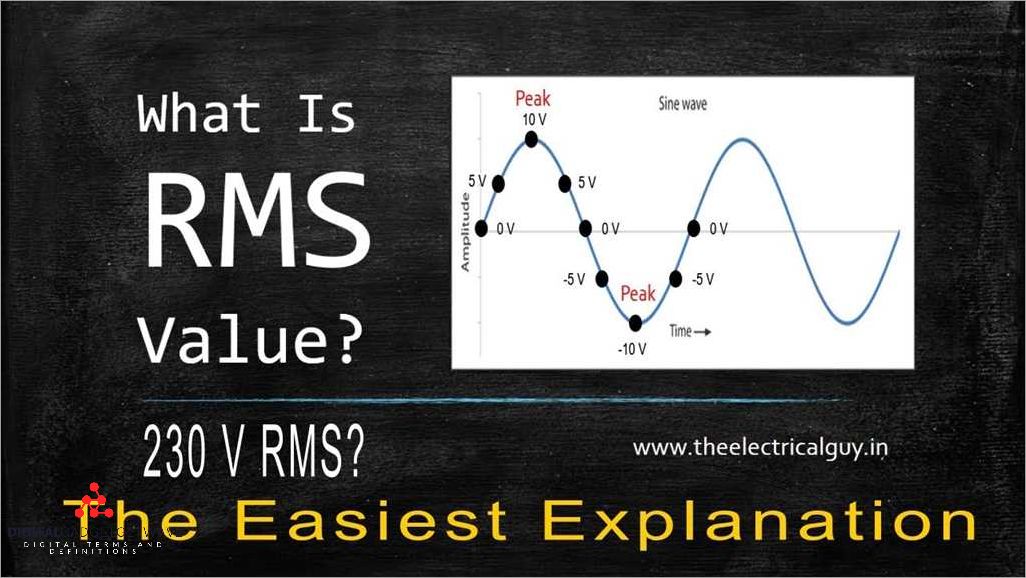
RMS, which stands for “Root Mean Square,” is a mathematical term commonly used in various fields to describe the average magnitude of a set of values. In essence, it is a way to measure the “typical” value of a set of numbers or data points. The concept of RMS has significant implications in fields such as statistics, physics, engineering, and signal processing.

In statistics, RMS serves as a measure of dispersion and is often used to calculate the standard deviation of a set of values. It provides insight into the variability or spread of the data. For example, if we have a set of measurements for a particular experiment, we can calculate the RMS to determine how closely the individual measurements align with the average value.

In physics, RMS is utilized to describe the intensity or amplitude of a waveform or signal. It is calculated by taking the square root of the arithmetic mean of the squares of the individual values. This enables scientists and engineers to quantify the energy or power associated with the signal, making it a valuable tool for analyzing and interpreting data.

In engineering, RMS is utilized in diverse applications such as electrical circuits, audio processing, and vibration analysis. It is a crucial parameter in understanding the behavior of systems and making informed decisions. For instance, in audio engineering, RMS is used to determine the average power or loudness of an audio signal, which helps in various tasks, including sound mixing and mastering.

Overall, the acronym RMS has a broad range of meanings and significance depending on the context in which it is used. Whether in statistical analysis, physics, engineering, or other fields, it provides a valuable quantitative measure that helps understand data, characterize signals, and analyze systems. Its applications are ubiquitous and essential in countless areas of study and industry.

Understanding RMS in Different Contexts

RMS, which stands for Root Mean Square, is a mathematical term that is commonly used in various fields to describe different concepts. It is essential to understand the context in which RMS is being used in order to fully grasp its meaning and significance.

In the field of mathematics and statistics, RMS refers to the square root of the mean of the squares of a set of numbers. It is a way of representing the average magnitude of a set of values, often used to measure variability or dispersion.

In the field of audio engineering, RMS is used to measure the average power of an audio signal, representing its overall loudness. It is particularly useful when dealing with complex waveforms that may have varying amplitude levels.

In the field of physics, RMS is used to calculate the effective value of an alternating current or voltage. It takes into account the amplitude and frequency of the waveform to determine its equivalent steady value.

In the field of electrical engineering, RMS is also used to quantify the power or voltage of an electrical signal. It is often used in calculations related to power consumption, electrical efficiency, and system performance.

RMS is also used in the context of software and technology industry to refer to a specific system or software development methodology known as “Requirements Management System”. It is a process used to define, track, and manage the requirements of a software project, ensuring that they are accurately documented and properly implemented.

Overall, the meaning and significance of RMS can vary depending on the specific context in which it is used. Whether it is in mathematics, audio engineering, physics, electrical engineering, or software development, understanding RMS is essential for professionals in these fields to effectively analyze and interpret data, measure signals, and ensure the successful completion of projects.

RMS in Physics and Engineering

RMS stands for “Root Mean Square” and it is a statistical measure used in physics and engineering to define the average value of a varying quantity. The root mean square is particularly useful for analyzing any time-dependent signals or fluctuating data that oscillate around a mean value.

The root mean square value is computed by taking the square root of the mean of the squared values of a data set. This calculation allows for the inclusion of both positive and negative values, making it suitable for data that fluctuates above and below zero. The RMS value provides a way to express the magnitude of an oscillating signal or the effective value of an alternating current or voltage.

In electrical engineering, the RMS value is commonly used to quantify the power of an alternating current or voltage. For example, when calculating the power of an AC circuit, it is important to use the RMS value of the current or voltage because it takes into account the varying nature of these signals.

The concept of RMS is also applied in the field of physics, especially in the analysis of waveforms. For instance, in studying sound waves or voltage signals, the RMS value is often used to describe the amplitude or intensity. It provides a meaningful representation of the average energy carried by these waveforms, allowing for easier comparisons and calculations.

In summary, RMS is a vital concept in both physics and engineering. It provides a statistical measure that helps in characterizing fluctuating data, analyzing time-dependent signals, and determining the effective values of alternating currents or voltages. Its application extends to various areas, including electrical engineering, telecommunications, acoustics, and signal processing.

RMS in Business and Finance

In the context of business and finance, RMS stands for Risk Management System. In today’s fast-paced and complex business environment, managing risk is crucial for the success and sustainability of businesses. A robust RMS plays a vital role in identifying, assessing, and mitigating potential risks that can impact the financial health and operations of a company.

Implementing an effective RMS involves a systematic approach that includes identifying and analyzing various types of risks such as financial, operational, legal, and reputational. It also involves developing strategies and processes to minimize or eliminate these risks. This may include implementing internal controls, insurance policies, contingency plans, and regular monitoring and reporting mechanisms to ensure risks are managed proactively.

A well-designed RMS not only helps businesses avoid financial losses but also enables them to seize opportunities and make informed decisions. By identifying potential risks and implementing appropriate risk mitigation measures, companies can protect their assets, enhance their reputation, and improve their overall financial performance.

RMS in Technology and Computing

RMS stands for “Root Mean Square” in the context of technology and computing. It is a mathematical term used to describe the way in which a set of values fluctuates around a certain average or mean value. The RMS value is calculated by taking the square root of the mean of the squares of a set of values. This calculation is commonly used in various fields of technology and computing, including electrical engineering, signal processing, and data analysis.

One of the most common applications of RMS in technology is in the measurement of electrical voltage. In this context, RMS is used to determine the effective voltage of an alternating current signal. The RMS value represents the equivalent direct current voltage that would produce the same amount of power as the alternating current signal. This is important in electrical engineering as it allows for accurate comparisons and calculations of power consumption and distribution.

In addition to electrical engineering, RMS is also relevant in the field of signal processing. It is commonly used to analyze and quantify the amplitudes of audio signals, such as music or speech. By calculating the RMS value of an audio signal, engineers can determine the overall energy content and level of a sound. This information is valuable in various applications, including sound recording, audio compression, and audio equalization.

In the field of data analysis, RMS is often used to measure the variability or dispersion of a set of data points. By calculating the RMS value, analysts can assess the average deviation of the data points from the mean value. This provides insights into the spread and distribution of the data, allowing for statistical comparisons and predictions. RMS is particularly useful when dealing with data sets that contain both positive and negative values, as it takes into account the magnitude of the deviations.

In conclusion, RMS stands for “Root Mean Square” in the context of technology and computing. It plays a crucial role in electrical engineering, signal processing, and data analysis. Whether it is used to measure electrical voltage, analyze audio signals, or quantify data dispersion, the RMS value provides valuable information and insights into various technological and computational processes.

The Importance of RMS Measurements

The term RMS stands for Root Mean Square, and it is a widely used measure in various scientific and engineering fields. RMS measurements are significant because they provide a way to assess the magnitude of electrical signals, especially those that are varying over time.

For example, in the field of electrical engineering, RMS measurements are crucial for understanding the power consumption and efficiency of electrical devices and systems. By calculating the RMS values of voltage and current signals, engineers can accurately determine the average power being consumed or generated by a device. This information is essential for designing and optimizing electrical systems, as well as for ensuring their safe operation.

The importance of RMS measurements also extends to other fields, such as audio engineering and signal processing. In audio engineering, RMS values are used to measure the amplitude of sound signals, which is crucial for determining the loudness or intensity of the audio. This information is vital for maintaining consistent sound levels in various audio applications, such as music production, broadcasting, and live performances.

Additionally, RMS measurements can be used to analyze and interpret data in various scientific experiments and research studies. In physics and mathematics, RMS values are frequently used to represent the average or typical value of a set of data points. By calculating the RMS values, researchers can gain insights into the variability and overall characteristics of the data, which can help drive further analysis and decision-making.

In conclusion, RMS measurements are important for assessing the magnitude and characteristics of electrical signals, sound signals, and other types of data. They play a critical role in various scientific and engineering fields and provide valuable information for designing, optimizing, and understanding systems and processes. Therefore, understanding and utilizing RMS measurements is essential for professionals in these fields to ensure accurate and efficient analysis and decision-making.

RMS in Sound and Audio

RMS, which stands for root mean square, is a mathematical concept widely used in the field of sound and audio. It is a measure of the average power or amplitude of a signal over a given time period. In sound engineering, the RMS value is often used to quantify the loudness or volume of a sound signal.

When a sound wave is represented as an electrical signal, it fluctuates in amplitude over time. The RMS value provides a way to measure the average amplitude of this fluctuating signal. This is important in audio applications as it allows us to quantify the signal’s power and determine its impact on speakers, amplifiers, and other audio equipment.

To calculate the RMS value of a sound signal, the signal needs to be squared, and then the mean (average) of the squared values is taken. Finally, the square root of the mean is found to obtain the RMS value. This method is used because squaring the values ensures that negative and positive values contribute equally to the average, taking into account the overall power of the signal.

The RMS value is particularly useful in audio production and editing to ensure that the overall sound is balanced and consistent. It helps to prevent distortion or clipping that can occur when the signal’s amplitude exceeds the capabilities of the audio equipment. By keeping the RMS within acceptable limits, sound engineers can ensure a high-quality and enjoyable listening experience.

In conclusion, RMS is a crucial concept in sound and audio. It provides a way to measure and quantify the average power or amplitude of a sound signal, allowing sound engineers to ensure the optimal performance of audio equipment and deliver a balanced sound experience to listeners.

RMS in Electrical Systems

The acronym RMS stands for “Root Mean Square” in electrical systems. It is a mathematical term that is used to calculate the effective value of an alternating current (AC) or voltage waveform. The RMS value is important in electrical engineering as it provides a way to measure and compare the power of different waveforms.

The RMS value is calculated by taking the square root of the mean of the squared values of the waveform. This mathematical technique allows engineers to find an equivalent direct current (DC) value that will deliver the same amount of power as the alternating current waveform.

One of the main reasons why the RMS value is significant in electrical systems is because it is directly related to the power delivered by AC sources. Power is calculated by multiplying the RMS value of the voltage by the RMS value of the current. Therefore, accurately measuring and calculating the RMS values of waveforms is crucial in determining power consumption and designing efficient electrical systems.

Another important application of the RMS value is in determining the operating limits and specifications of electrical devices. The RMS value of a waveform can help determine the maximum voltage or current that a device can handle without being damaged. This information is crucial in designing and selecting appropriate components for electrical systems.

In conclusion, the RMS value plays a significant role in electrical systems. It allows engineers to accurately measure and compare waveforms, calculate power consumption, and determine the operating limits of electrical devices. Understanding and utilizing the concept of RMS is essential in designing efficient and reliable electrical systems.

RMS in Data Analysis and Statistics

RMS stands for Root Mean Square, which is a commonly used statistical measure in data analysis. It is a mathematical calculation that provides a way to summarize and interpret data by taking into account both the spread and magnitude of values within a dataset. The RMS value is particularly useful in fields such as finance, physics, and engineering, where accurate and precise measurements are crucial for making informed decisions and predictions.

When working with data analysis and statistics, the RMS value is often used as a measure of variability or dispersion. It allows researchers and analysts to assess the amount of variation or fluctuation present in a dataset. This is especially important when dealing with time series data or any data that exhibits periodic or cyclical patterns.

The RMS is calculated by taking the square root of the arithmetic mean of the squared values of a dataset. This method allows for the cancellation of positive and negative values, providing a positive and more representative value that accurately reflects the distribution and amplitude of the data points.

The RMS value is used in various statistical techniques and models, such as regression analysis, Fourier analysis, and signal processing. It helps in identifying trends, understanding the magnitude of fluctuations, and estimating the overall magnitude or strength of a signal or phenomenon. By utilizing the RMS value, analysts can make more accurate predictions and draw meaningful insights from data.

In conclusion, the RMS value plays a significant role in data analysis and statistics. It is a powerful tool for measuring variability and assessing the magnitude of data points. Its application in various fields underscores its importance in making sound decisions and drawing meaningful conclusions from data.

Common Misconceptions about RMS

RMS stands for Root Mean Square is a common misconception. While it is true that RMS does stand for Root Mean Square, it is important to understand that this term is not exclusive to electrical engineering. The concept of RMS is widely used in various fields, including mathematics, statistics, physics, and signal processing.

Another common misconception is that RMS is only used to measure the amplitude of a periodic signal. While RMS is commonly used in the context of measuring the amplitude of a signal, its application goes beyond that. RMS is also used to calculate the average power of a signal, as well as to measure the effective value of a varying signal.

There is also a misconception that RMS is the same as peak value. This is not accurate. The peak value of a signal represents its maximum instantaneous value, while RMS represents the equivalent DC value that would produce the same power dissipation in a resistive load. In other words, the peak value and RMS value are related, but they serve different purposes.

RMS is not an accurate representation of the entire signal is another misconception. While it is true that RMS focuses on the amplitude or power of a signal, it still provides valuable information about the signal. By calculating the RMS value, one can characterize the overall magnitude of a signal, which can be useful in various applications.

RMS is only relevant in electrical engineering is a misconception that limits the understanding of this concept. As mentioned earlier, RMS is used in various fields. For example, in statistics, RMS is used to measure the spread or deviation of a set of values from their mean. Understanding the broader application of RMS can help to gain a deeper appreciation for its significance.

In conclusion, while RMS does stand for Root Mean Square, it is not limited to electrical engineering, it is not the same as peak value, it provides valuable information about a signal, and it has broader applications beyond amplitude measurement. Understanding these misconceptions can lead to a more accurate understanding and application of RMS in various fields.

RMS vs. Peak Values

When it comes to measuring electrical signals, two important values to consider are RMS and peak values. RMS stands for Root Mean Square, while peak values represent the maximum amplitude of a signal.

The RMS value is a statistical measure that calculates the square root of the average of the squares of the values in a signal. It provides a more accurate representation of the true power of the signal, especially in the case of AC (alternating current) signals. The RMS value takes into account both the positive and negative peaks of the signal, providing a more balanced measurement.

The peak value, on the other hand, simply represents the highest value the signal reaches. It does not consider the negative portion of the signal, focusing only on the positive amplitude. Peak values are commonly used when measuring the instantaneous magnitude of a signal or when determining the maximum capability of a system.

While the peak value can provide valuable information, it is important to note that it does not accurately represent the overall power or energy of a signal. RMS values, on the other hand, provide a more meaningful measure of the true power being delivered by the signal. Therefore, when comparing and analyzing signals, it is generally more appropriate to use RMS values.

In summary, RMS and peak values are both important in understanding and measuring electrical signals. While peak values represent the maximum amplitude of a signal, RMS values provide a more accurate representation of the true power being delivered. When analyzing signals and determining their power or energy, it is generally recommended to use the RMS values for a more meaningful measurement.

RMS vs. Average Values

When discussing electrical signals, it is important to understand the difference between RMS (Root Mean Square) values and average values. While both values provide information about an electrical signal, they serve different purposes and offer different insights into the signal’s behavior.

RMS stands for Root Mean Square and is a statistical measure used to calculate the effective value of an alternating current or voltage signal. It represents the magnitude of the signal in terms of its power, taking into account the fluctuations and varying amplitudes of the signal over time. RMS values are particularly important for analyzing sinusoidal signals, as they accurately represent the power or energy associated with these types of signals.

For example, when calculating the RMS value of a sinusoidal signal, we take the square root of the average of the squares of the signal’s amplitude over one period. This allows us to obtain a value that is directly proportional to the power of the signal, making it useful for various calculations and comparisons.

Average values, on the other hand, represent the arithmetic mean of a set of values. In the context of electrical signals, average values are often used to calculate the average power or voltage over a given time period. Unlike RMS values, average values do not consider the fluctuations and varying amplitudes of the signal, but rather provide a simple representation of the signal’s behavior over a specified duration.

For example, when calculating the average value of an AC signal, we add up all the instantaneous values of the signal over a defined time period and divide the sum by the length of that period. This gives us an average value that provides information about the signal’s average behavior over that time frame, but does not capture the signal’s fluctuations or power accurately.

In summary, RMS values and average values both have their unique significance when analyzing electrical signals. While RMS values provide a more accurate representation of the signal’s power or energy, average values offer insights into the signal’s behavior over a specific time period. Understanding the difference between these values is crucial for accurate analysis and interpretation of electrical signals.

RMS vs. Root Sum Square (RSS)

RMS stands for Root Mean Square, while RSS stands for Root Sum Square. Both of these terms are commonly used in statistics and mathematics to describe methods of calculating the average of a set of values.

The RMS is a statistical measure that calculates the square root of the average of the squared values in a set. It is often used to measure the magnitude of a variable or the average value of a waveform. The RMS value provides a more accurate representation of the data compared to other average calculations, as it takes into account the amplitude of each value.

On the other hand, the RSS is a mathematical method that calculates the square root of the sum of the squared values in a set. It is commonly used in engineering and physics to calculate the overall magnitude or error of a system composed of multiple variables or components. The RSS value provides a measure of the combined effects of these variables or components.

While both the RMS and RSS involve squaring and taking the square root of a set of values, they serve different purposes. The RMS is used to calculate the average value or magnitude of a single variable, while the RSS is used to calculate the overall magnitude or error of a system composed of multiple variables.

In summary, the RMS and RSS are both mathematical methods used to calculate average values or magnitudes, but they have different applications. The RMS is used for single-variable calculations, while the RSS is used for multi-variable calculations. Understanding the differences between these methods is important in various fields of study, such as statistics, engineering, and physics.